PDF

Int J Biol Sci 2011; 7(1):102-111. doi:10.7150/ijbs.7.102 This issue Cite

Research Paper

Local Rheology of Human Neutrophils Investigated Using Atomic Force Microscopy

Yong J. Lee¹, Dipika Patel², Soyeun Park²

1. School of Mechanical Engineering, Kyungpook National University, Daegu, South Korea
2. Department of Physics, Texas Tech University, Box 41051, Lubbock, TX 79409

✉ Corresponding author: phone: 1-806-742-2264, fax: 1-806-742-1182, email: soyeun.parkedu

Abstract

During the immune response, neutrophils display localized mechanical events by interacting with their environment through the micro-vascular transit, trans-endothelial, and trans-epithelial migration. Nano-mechanical studies of human neutrophils on localized nano-domains could provide the essential information for understanding their immune responsive functions. Using the Atomic Force Microscopy (AFM) - based micro-rheology, we have investigated rheological properties of the adherent human neutrophils on local nano-domains. We have applied the modified Hertz model to obtain the viscoelastic moduli from the relatively thick body regions of the neutrophils. In addition, by using more advanced models to account for the substrate effects, we have successfully characterized the rheological properties of the thin leading and tail regions as well. We found a regional difference in the mechanical compliances of the adherent neutrophils. The central regions of neutrophils were significantly stiffer (1,548 ± 871 Pa) than the regions closer to the leading edge (686 ± 801 Pa), while the leading edge and the tail (494 ± 537 Pa) regions were mechanically indistinguishable. The frequency-dependent elastic and viscous moduli also display a similar regional difference. Over the studied frequency range (100 to 300 Hz), the complex viscoelastic moduli display the partial rubber plateau behavior where the elastic moduli are greater than the viscous moduli for a given frequency. The non-disparaging viscous modulus indicates that the neutrophils display a viscoelastic dynamic behavior rather than a perfect elastic behavior like polymer gels. In addition, we found no regional difference in the structural damping coefficient between the leading edge and the cell body. Thus, we conclude that despite the lower loss and storage moduli, the leading edges of the human neutrophils display partially elastic properties similar to the cell body. These results suggest that the lower elastic moduli in the leading edges are more favorable for the elastic fluctuation of actin filaments, which supports the polymerization of the actin filaments leading to the active protrusion during the immune response.

Keywords: Atomic force microscopy, neutrophils, micro-rheology, viscoelasticity, nano-indentation, Young's modulus

Introduction

The neutrophils are the most abundant type of white blood cells, comprising about 50-70% of all white blood cells. They play a primary role in protecting the human body from the infection through phagocytosis, i.e., ingestion. Responding to infectious and inflammatory signals, neutrophils quickly migrate to the site of infection and inflammation through the micro-vascular transit, trans-endothelial, and trans-epithelial migration [1, 2]. During this process, the neutrophils continuously undergo adhesion, detachment, deformation, recovery, extension, and contraction. These mechanical events require the active rearrangements of actin cytoskeleton, a three-dimensional polymeric internal structure [3, 4]. The actin cytoskeleton is known to consist of actin filaments, microtubules, and intermediate filaments with many accessory proteins. The dynamic reassembly of this intracellular protein network regulates the cell shape, cellular adhesions to the extracellular matrix, and mechanical compliances, i.e., elastic moduli [5, 6]. Such mechanical events are orchestrated to generate cells' directional movements. Thus, the elastic properties of the neutrophils are strongly associated with their immune responsive functions through mechanically mediated signaling pathways. The neutrophils often circulate through the micro-capillaries with a diameter smaller than their own size [7, 8]. They also migrate and penetrate through various tissues such as endothelial and epithelial tissues [9]. These processes involve the dynamic deformation and recovery over a wide time scale range. Thus, it is of critical importance to accurately investigate rheological properties, i.e., visco-elastic properties in addition to quantifying static elastic moduli of the neutrophils. The rheological studies of the neutrophils should provide vital information in understanding the cascading mechanism for pathological changes initiated by the neutrophils. Recent studies suggest that the complications of diabetes mellitus can be attributed to the altered lymphocyte stiffness [10] and the decreased deformability of leukemia cells can be highly linked to leukostasis [11].

Many of the past rheological techniques were developed to investigate the viscoelastic properties of the whole cell in suspension. Global techniques such as the micropipette aspiration, optical tweezers, and the optical stretchers were used to characterize the time-dependent mechanical behaviors of a single cell [12-17]. Especially, the micropipette aspiration technique was extensively used to investigate the rheological properties of the neutrophils [12, 18]. In this technique, the protrusive evolution of a neutrophil aspirated into the micropipette was monitored as a function of time to deduct the viscoelastic properties by considering the resistance of the neutrophil protrusion to a micropipette followed by liquid flow [19, 20]. It provides some valuable information when the entire cell undergoes a deformation as the cell moves through a relatively large capillary. Nevertheless, the obtained information was not enough to quantitatively describe localized viscoelastic properties within a cell [21]. Optical bead micro-rheology based on laser-interferometry and magnetic twisting cytometry were applied to investigate the viscoelastic properties of the neutrophils in localized domains [22, 23]. These techniques provided low stress information over a wide range of frequency (0.1-100 Hz). However, the measurement points were limited to the binding locations of the magnetic beads, and the mechanical properties of the neutrophils became altered due to the attachment of the magnetic beads to the cell membrane [23]. Although the optical bead micro-rheology showed a regional variation in the viscoelastic moduli, the reported moduli differed from the values previously reported from the global measurement techniques by two orders of magnitude [22]. Thus, a more accurate measurement is required to investigate the variation in the viscoelastic moduli in local domains within a single neutrophil. The neutrophils undergoing the micro-vascular transit, trans-endothelial, and trans-epithelial migration during the immune response involve highly localized mechanical events such as adhesion and deformation. The ability for local measurements with micron or submicron lateral resolution is needed to study the localized mechanical events.

A nano-indentation technique using Atomic Force Microscopy (AFM) has advantages over other previously mentioned methods [24]. The AFM has been used to investigate the nano-mechanics of various cell types in localized cell domains with a nanometer precision. It can be also extended to the localized rheological measurements over a wide frequency range [25]. However, measurable contributions from the underlying hard substrate on which a cell is placed and a high stress produced by the sharp AFM tip hindered accurate measurements in thin regions of the cells such as the leading edge, i.e., the lamellipodium. Recent studies using a modified spherical tip have achieved a controlled non-destructive stress (100 Pa - 10 kPa) on the cell under investigation [26]. In our previous studies using fibroblasts, we have expanded the applicable regions to the thin leading edge by adapting more advanced analytical models that consider the thinness of the sample, the hard substrate effect, and the high strain [27]. Two different models - the Chen and Tu models -were developed for thin samples, depending on the adherent properties of a cell on a hard substrate. While the Chen model was applied in regions where cells adhere well onto the substrate, the Tu model considers the freely sliding regions. The AFM-based nano-indentation technique has become more powerful when used as a micro-rheological tool. Previously, we have quantified the frequency-dependent viscoelastic moduli of biological cells by adding a sinusoidal oscillation with a small amplitude on the AFM's z-scanner while taking the force-distance curves [28]. The in-phase and out-of-phase responses obtained with the lock-in modulation technique reflect the viscoelastic properties of cells as storage and loss moduli. This technique is applicable over a wide range of frequencies (100 to 300 Hz).

In this paper, we investigate the local variation in viscoelastic moduli of the human neutrophils using the AFM-based micro-rheology. The human neutrophils were isolated from the human blood and seeded onto the glass slides. When the neutrophils firmly adhere, the force-distance curves were taken from localized domains to investigate local variations in the elastic moduli. The standard Hertz and more advanced Chen and Tu models were applied to calculate the elastic moduli from different regions. The viscoelastic moduli were also determined from the frequency-dependent measurements. The models applied for the static nano-indentation measurements were extended in order to obtain the viscoelastic moduli from the frequency-dependent measurements.

Methods

Human neutrophil preparation

The data were taken from the human neutrophils isolated from the human whole blood using a density gradient technique described in detail elsewhere [29]. Briefly, 7.5 ml of peripheral blood were drawn from healthy volunteers with heparinized syringes. The blood was added to the equal amount of commercially available mono-poly resolving medium, Lympholyte®-poly (Cedarlane laboratories limited, Ontario, Canada) and centrifuged. After the centrifugation, the poly-morphonuclear cells were harvested from the lower band using a Pasteur pipette. The harvested cells were diluted with an equal volume of 0.45% saline solution in order to restore the normal osmolality and washed twice with PBS. For the AFM experiments, cells were re-suspended in the Ca-/Mg-free blood buffer (5 mM of KCl, 20 mM of HEPES, 100 mM of NaCl, 25 mM of Glucose, 0.1 mM of Adenine, 0.1 mM of Inosine, 0.5% of Albumin, 5 units/ml of Heparin, and 1% of antibiotics) [30, 31]. The solution of the blood buffer containing from 10⁵ to 5×10⁵ cells was then placed on the pre-sterilized cover-slip at 10 minutes before data were taken. For the measurements, well-adhered and motile neutrophils were selected. During AFM measurements, we continuously provided a small amount of the medium solution to the human neutrophils through the inlet of the liquid AFM cell (Microcell, TM Microscope, Sunnyvale, CA and Bioheater^TM, Asylum Research, Santa Barbara, CA). In order to ensure the viability of investigated cells, the data collection was carried out only for the first two hours after starting the measurements.

AFM measurements

All AFM measurements were taken with a MFP 3D® (Asylum Research, Santa Barbara, CA) and an Autoprobe CP (TM Microscope, Sunnyvale, CA) at room temperature. A polystyrene bead (Seradyn Particle Technology, Indianopolis, IN) - glued to commercial cantilevers (Microlevers, Veeco Probes, Camarillo, CA) were used in order to obtain a well-defined contact area and to reduce the stress from otherwise sharp AFM tips [28]. The cleanliness and the radius of the modified probe tip (1.5-4 μm) were verified by the reverse AFM topographic image [27]. The force constant of each cantilever (0.02-0.06 N/m) was calibrated against a cantilever with the known force constant. The calibration cantilever was selected when the force constants from three different calibration methods agreed within 5% [27]. The three methods involve the calibration with a commercial cantilever of known force constant (0.157 N/m, TM Microscopes), the thermal fluctuations method, and the resonance frequency method. The nano-indentation experiments where the force curves were acquired with a one-second time interval, i.e., 1 Hz, were performed in order to obtain the static elastic moduli. The frequency-dependent viscoelastic moduli were obtained by superimposing a sinusoidal drive signal with small amplitude (2-5 nm) and high frequency (50-300 Hz) on the AFM's z-scanner while taking the force curves. The lock-in modulation technique was used to monitor the in-phase and out-of-phase components of the tip-sample interaction force. As a control experiment, a force curve was obtained on a glass slide before being taken on the cells to make sure of the linear increase in force on the hard substrates as a function of the scanner displacements. The experimental details are described in our previous publication [28].

Data Analysis

Static elastic moduli

The force on a neutrophil f and the indentation δ were used to generate f-δ curves by calculating the indentation and the force from the obtained scanner displacements and cantilever deflections. The indentations δ were calculated as the subtraction of the cantilever deflections from the scanner displacements. The forces f applied on the neutrophils were calculated by multiplying the force constant k with the cantilever deflection. The contact point between the tip and the sample was determined as the point where the slope of the force curve initially deviates from zero. First, the Hertz model was applied to determine the elastic moduli from f-δ curves. According to the analytical expression for the Hertz model (Eq.1), the f-δ curve is converted to the curve of elastic constant K =E/(1-ν²) versus the dimensionless quantity δ/R, where R is the radius of the spherical tip and ν is the Poisson ratio. The elastic constant K remains nearly constant as δ/R varies as would be expected for linear homogenous samples.

 (1) 

However, in thin regions such as the lamellipodia, the Hertz model is no longer valid due to the strong substrate effect. In these regions, the data are better analyzed with the Tu and the Chen models, which are modified from the Hertz model by considering the hard substrate effect [27]. The different boundary condition was applied for each model; the well-adhered conditions for the Chen model and the freely sliding conditions for the Tu model. For these models, the cell's height h at the measurement point was determined from the AFM topographic images. The inevitable slight indentation occurring in the contact mode imaging was extrapolated from the force curve and added to the height. The dimensionless quantity δ/R for each force curve was converted to the new dimensionless quantity δR/h². The elastic constant K of the Hertz model was converted to the new constant for the Chen and Tu models. The details of this procedure are described elsewhere [27]. We choose the elastic constant from the model that gives the least variation in the elastic constants as δR/h² is varied because we assume that the cells are modeled as a linear material.

Frequency-dependent viscoelastic moduli

For the frequency-dependent measurements, the total indentation is the sum of the oscillating indentation due to the oscillating scanner displacement and the offset indentation δ₀ from the slowly varying scanner displacement. In this case, the contact point is determined as the point at which the maximum change in the phase difference between the cantilever and scanner signals occurs. The cantilever before contact is subject to the hydrodynamic drag of the surrounding viscous medium leading to a constant phase difference close to 90 degrees. Once the tip makes contact with the sample, the phase difference significantly decreases. This drag force was directly measured by monitoring the cantilever oscillation just before the tip makes contact with the sample and subtracted from the total oscillatory force. The elastic constant K'=E'/(1-ν²) and viscous constant K” =E”/(1-ν²) are obtained by expanding the oscillating deformation force 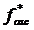with respect to the indentation δ as a Taylor series in a complex form (Eq.2).

 (2) 

The in-phase real and the out-of-phase imaginary components reflect the elastic (storage) and the viscous (loss) constants of the sample, respectively. Analogous to the nano-indentation experiments, in thin regions, the Tu and the Chen models were applied appropriately. The elastic and viscous constants were determined from the model that shows the least variation in K' or K” as δR/h² is varied. Detailed calculations needed for both models are described elsewhere [27, 28].

The frequency dependence of K' and K'' were analyzed by the structural damping law; K' ~ ω^x-1, where ω is the angular frequency [32, 33]. Briefly, the power law exponent x-1 was calculated from the power law fit to the data of K' or K” versus ω. Because we used the relatively low frequencies (50 - 300 Hz), the structural damping coefficient, i.e., hysteresivity η was calculated as K”/K' for the given frequency. For the comparison, the corresponding power law exponent were calculated from the structural damping coefficients using η=tan((x-1)π/2).

Results

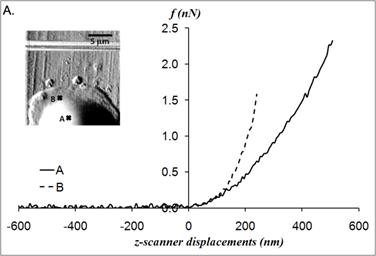
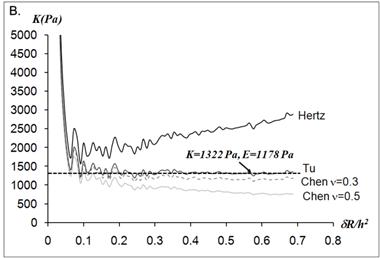
The static elastic moduli were measured at three different points on each neutrophil to characterize local variations in the elastic moduli. Typical force versus z-scanner displacements curves (see Fig. 1A) were obtained from the following three points: points closer to the body, near the leading edge, and near the tail. While the elastic moduli from regions closer to the cell body were best analyzed with the Tu model (Fig. 1B), the data obtained from the regions closer to the leading edge were better analyzed with the Chen model (Fig. 1C). The Hertz model failed to adequately describe the obtained data from both points in Fig. 1 because the substrate effect dominates the elastic behavior in such thin regions of the cell. The Chen and the Tu models adapt the two distinctly different interfacial properties. The Chen model was developed to describe the thin layer rigidly adhered to the substrate. A strong adhesion between the cell membrane and the substrate provides an additional support for the cell to resist lateral deformations that release the energy. On the other hand, the Tu model describes the non-adhered layer, which slides freely on the substrate while the substrate opposes only vertical deformations. Thus, the data shown in Fig.1 implies that the thin regions relatively near the leading edge of the neutrophils strongly adhere to the substrate while the regions closer to the cell body lose their adherence. These results were consistent with the previous findings on the mouse fibroblasts [27, 28].

 Figure 1 

(A) Force versus z-scanner displacement curves obtained from the points indicated in the AFM deflection images shown in the inset. (B, C) The static elastic constants K versus δR/h² as calculated from the Hertz, Tu, and Chen models with various Poisson ratios ν. The heights of the measurement points h were measured from the AFM topographic image. The best result is obtained (B) with the Tu model at point A (h_A=1,571 nm) and (C) the Chen model with the Poisson ratio of 0.5 at point B (h_B=356 nm).

The average elastic moduli E determined from ten different neutrophils are shown in Fig. 2. The statistical analysis was performed to determine whether the static elastic moduli vary in different morphological regions - regions closer to the body, the leading edge, and the tail - of the human neutrophils. As plotted in Fig. 2, the elastic moduli in the regions closer to the cell body were significantly higher than those in the regions closer to the leading edge (686 ± 801 Pa) and the tail (494 ± 538 Pa). However, the leading edge and the tail were not mechanically different from each other. Thus, from the statistical analysis of the static elastic moduli determined from the local domains of the human neutrophils, we found that the static elastic moduli increase from the leading edge to the cell body. This indicates that the leading edges of the human neutrophils are mechanically more compliant and softer compared to the cell body. This local profile of a human neutrophil is consistent with the observations made from the mouse fibroblasts - NIH3T3 and BALB3T3 fibroblasts - placed on the glass substrates [28].

 Figure 2 

Static elastic moduli E locally observed from the three distinctly different regions of the human neutrophils. The three different regions of a human neutrophil were selected as the regions closer to the body, the leading edge, i.e., lamellipodium, and the tail. Ten different neutrophils were investigated. The lamellipodium and the tail display a similar elastic behavior, but the regions closer to the body display higher elastic moduli compared to the regions closer to the leading and the tail. * and ** indicate the statistical significances of p < 0.05 and p <0.01, respectively.

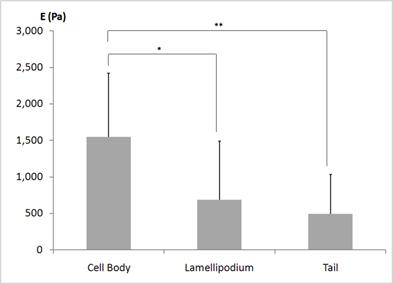
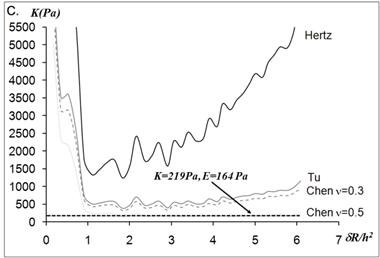
As indicated by the large standard deviations in Fig. 2, we observed a wide distribution in static elastic moduli throughout the cell body. Many previous AFM-indentation experiments have been performed in the cell body in order to avoid the hard substrate effect and complicated analysis needed if the Hertz model cannot be used. In these studies, force curves were obtained by positioning the AFM tip over the central region of a cell by monitoring the bright-field microscopic images [32, 34]. It is considered that the elastic moduli obtained from the relatively thick cell body region do not suffer from the overestimation due to the hard substrate effect. In addition, the localized investigation over the central region of a cell was more favorable in studies comparing the mechanical properties of different cell lines. However, we found that the determined elastic moduli have a large variation although the measurement points were all located in the cell body. An extreme example is presented in Fig. 3.

 Figure 3 

Static elastic constants K determined from three different nano-domains of a human neutrophil. All measurement points - A, B, and C - were located in the regions closer to the cell body, as indicated in the AFM deflection image shown in the inset. While the elastic constant at point A was obtained from the Hertz model, the Chen model with the Poisson ratio of 0.5 was needed to determine the elastic constants at point B and C. The height of each measurement point did not differ much from each other; h_A = 1,936 nm, h_B = 2,008 nm, and h_C = 1,622 nm.

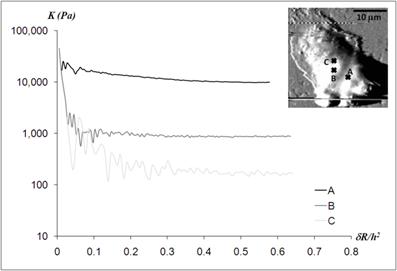
The three measurement points were constrained to the central region of a human neutrophil as indicated in the AFM deflection mode. The heights of all three measurement points were relatively thick compared to those in the leading edge of the cell; 1,936 nm, 2,008 nm, and 1,622 nm for the point A, B, and C, respectively. While the Hertz model was applicable to obtain the static elastic moduli at the point A, the Chen model with the Poisson ratio of 0.5 was required to deduct the static elastic moduli from the force curves obtained at the point B and C. The Young's moduli were determined as 10,870 ± 254 Pa, 657 ± 27 Pa, and 124 ± 12 Pa for the point A, B, and C, respectively. Although all measurement points were in the close proximity (less than 5 μm), the static elastic moduli varied by two orders of magnitude. While the large variations in the static elastic moduli of the cell body came from different points within the same cell, the large variations of the lamellipodia and tails shown in Fig.2 were mainly attributed to the cell-to-cell variations.

Similar to the static nano-indentation data in thin regions such as the leading edge, the frequency-dependent data were also better analyzed with the Tu or the Chen model with a Poisson ratio ν of 0.4 or 0.5. Typical frequency-dependent data from the AFM-based micro-rheology are presented in Fig. 4.

 Figure 4 

Elastic |E'| and viscous |E”| moduli, plotted as a function of modulation frequency (100 Hz - 300 Hz) for a human neutrophil. The elastic (viscous) moduli are shown by the solid (dotted) line. The data were taken at the points indicated in the AFM deflection image shown in the inset. The point A is located near the leading edge and the point B closer to the cell body. Over the studied frequency range, the elastic components are greater than the viscous components, indicating a partial rubber plateau region (|E'|>|E”|) at both measurement points. The elastic moduli of the point A are greater than ones of the point B at the corresponding frequency.

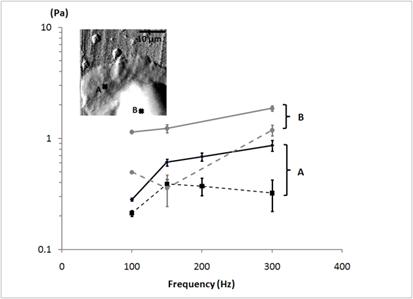
The frequency-dependent elastic moduli |E'| are comparable to the static elastic moduli E. In Fig. 4, the storage |E'| and loss moduli |E”| obtained from a human neutrophil are plotted as a function of the frequency, The measurement points were selected from the leading edge (the point A in Fig.4) and the region closer to the cell body (the point B in Fig.4) as indicated in the AFM deflection image. At both measurement points, the human neutrophil displays a partial rubber plateau where the elastic moduli |E'| were slightly greater than the non-disparaging viscous moduli |E”| for a given frequency within the observed frequency range (100-300 Hz). The human neutrophils do not display a purely elastic behavior but they display the visco-elastic dynamic behavior. We also found a significant frequency dependence of viscoelastic moduli from the human neutrophils. We observed the increase in the elastic (viscous) moduli as the modulation frequency increases. As presented in Fig. 4, the data obtained from the cell body (the point B) shows the power exponent of 0.46 in structural damping theory. This is consistent with the previous AFM-based micro-rheology studies on fibroblasts and polymer gels displaying the structural damping behavior with a power exponent of 0.5 [26]. The elastic moduli were nearly doubled for a four-fold frequency increment.

 Figure 5 

The average elastic |E'| and viscous |E”| moduli determined locally in the cell body and in the lamellipodium of the human neutrophils for the modulation frequency of 100 Hz. Fifteen different human neutrophils were investigated. One point in the cell body and the other point in the lamellipodium of each neutrophil are included in the statistical analysis.

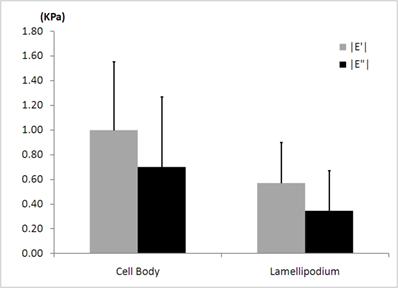
As shown in Fig. 5, the average elastic moduli |E'| (998 ± 556 Pa) obtained in the cell body of human neutrophils at the frequency of 100 Hz, agree well with the reported values of mouse neutrophils by the AFM [32]. We observed that there are about 40% decreases in both the average elastic |E'| and viscous |E”| moduli in the leading edge of the human neutrophils when compared to those in the cell body. The regional difference in average elastic moduli obtained by the rheological measurements resembles the case for the static elastic moduli determined in the local nano-domains. We also found that the average viscous moduli |E”| (700 ± 569 Pa) obtained in the cell body of the human neutrophils at the frequency of 100 Hz were slightly smaller than the average of elastic moduli |E'|, indicating not purely elastic but visco-elastic behavior. The structural damping coefficients, i.e., hysteresivities, were measured to be 0.7 in the cell body and 0.6 in the lamellipodium for the frequency of 100 Hz. These coefficients correspond to the power exponents of 0.39 and 0.34 from the structural damping theory, again indicating not purely elastic but visco-elastic properties in both regions [35].

Discussion

We observed the static elastic moduli of 1,548 ± 871 Pa in the cell body of the human neutrophils, which agree with the previously reported values from the rat neutrophils using the AFM [22]. However, these results are an order of magnitude higher than the globally determined elastic moduli of the neutrophils by aspirating them into the tapered micropipettes [19]. This discrepancy may rise from the methodological difference. The soft actin cortex or the liquid-like compartment of the cytoplasm contributes to the global deformation of a neutrophil when aspirated to the micropipette and results in the underestimation of the elastic moduli. However, the micro-domains of the densely packed structures would play a major role in the stress-strain response by the AFM indentation. This discrepancy between the global and local measurements may reflect the organizational variation of the actin cytoskeleton between the suspended and adherent neutrophils.

The consistent results from our measurements using more advanced models and previous AFM study using only the Hertz model suggest that the Hertz model is adequate for determining the elastic moduli from the relatively thick cell body. Interestingly, we also observed the mechanical heterogeneity within a neutrophil as shown in Fig. 3. There are many strong evidences that a cell is highly compartmentalized, and the organization of the actin cytoskeleton varies according to the actin filament density, the degree of cross-linking, and the formation and density of focal adhesions, i.e., the nexus between the basal cell membrane to the adherent substrate. The recent theoretical calculations showed that a cell's elastic modulus can differ by an order of magnitude depending on the degree of cross-linking of the actin filaments, the concentration of actin-binding proteins, and the presence of actin stress fibers [36]. Moreover, the central region of a cell also includes the nucleus, which leads to a large increase in the elastic moduli. The recent findings on human mesenchymal stem cells revealed that the nucleus is responsible for the high elastic moduli (54.3 ± 37.4 kPa) [37]. These findings suggest that the wide variation in the elastic moduli presented in Fig. 3 could have been resulted from the highly localized arrangement of the actin cytoskeleton and other cellular compartments. Although our analysis treats a neutrophil as a linear material, the wide distribution in the measured elastic moduli from the human neutrophils reflects that the human neutrophils are highly compartmentalized and their actin cytoskeletons form a heterogeneous organization.

Previously, mechanical studies using the AFM on the neutrophils were limited to the thick cell body where the Hertz model was applicable. The thin regions such as the leading edges of the neutrophils were not accessible with the AFM studies due to the hard substrate effect in the thin regions [32, 34]. By using the Tu and the Chen models, we were able to investigate thin regions as well. Thus, we were able to investigate the local variation in elastic moduli over the different regions of the neutrophils including the lamellipodium, which plays a critical role in understanding the motile behavior of the human neutrophils during the immune response [21]. According to the statistical analysis for the regional static elastic moduli, the lamellipodium and the tail of the human neutrophils display significantly lower elastic moduli compared to the cell body. This regional variation in elastic moduli of a human neutrophil is identical to the spatial profile of the elastic moduli delineated from mouse fibroblasts [26]. Similar to the motile fibroblasts, this result suggests that the lamellipodial protrusion, responsible for the active motile behavior and the dynamic deformation of the human neutrophils during the immune response, could be activated by the elastic fluctuation of the actin filaments [38, 39]. However, many details await further investigation in order to be conclusive in this molecular picture.

In addition, more advanced models resolving the hard substrate effect allow us to determine the adhesiveness of the local domains of the human neutrophils on the substrates. The Tu and Chen models consider the two distinctly different boundary conditions - well-adhered and freely-sliding layers on the substrate. Thus, by determining the best-fitted model, we found that the regions near the leading edge is well adhered but the regions closer to the cell body is poorly adhered to the substrates (Fig. 1). This result is consistent with adhesive properties found from the motile fibroblasts [28]. The motile cells are known to develop denser focal adhesions in the leading edge to support their protruding movements but the regions further away from the leading edge have less focal adhesions.

The frequency-dependent measurements provide more detailed information. Over the observed frequency range (100-300 Hz), the human neutrophils display the partial rubber plateau where the elastic moduli are greater than the viscous moduli. This rheological finding, consistent with previous studies on the fibroblasts and neutrophils using the AFM and optical rheology, implies that the mechanical response of a human neutrophil is dominated by the actin cytoskeleton [22, 26-28]. According to the soft glassy rheology, the cell's mechanical state varies between an elastic solid and a viscous liquid, corresponding to a power exponent between zero and one [35]. Recent rheological studies using the optical rheology and the AFM reported a power law structural damping behavior of the adherent neutrophils. We also observed the similar damping behavior in the regions closer to the cell body of the human neutrophils. However, there was a large discrepancy in the reported structural damping exponents depending on the utilized methodology. While the optical rheological studies reported 0.5 for the power exponent for the cell body of the crawling neutrophil, the AFM and magnetic twisting cytometry reported much smaller power exponents of 0.15 and 0.20, respectively [15, 22, 23, 32, 40]. This discrepancy implies that different measurement techniques are sensitive to the different cellular components contributing to the elastic moduli. It can be postulated that the liquid-like cytoplasm is the major component contributing to the elastic moduli measured by the optical rheology while the actin-rich cortex might account for the mechanical response in AFM and magnetic twisting cytometry. Interestingly, we found the power exponent of 0.46 in the cell body of the human neutrophils (see the data taken at the point B in Fig. 4), which agrees better with the optical rheological studies. However, the wide variations in the storage and loss moduli and the relatively narrow frequency range result in the power exponent of 0.98 in the leading edge, which is not comparable to the previously reported values for either polymer gels or biological cells (see the data taken at the point A in Fig. 4). Within our observed frequency range, it is difficult to compare the regional differences in the power exponent. It is required to obtain the viscoelastic information in a much wider frequency range in order to determine the power exponent from the structural damping theory. Despite the non-realistic quantitative measurement of the power exponents, these regional differences in the structural damping behavior are qualitatively consistent with the previously reported data using the optical rheological techniques. They reported a higher power exponent in the leading edge, implying more fluidic behavior compared to the elastic cell body [22]. This result is also consistent with the lower elastic moduli in the leading edge determined from the static nano-indentation measurements. From the viscoelastic moduli determined at 100 Hz, we found the structural damping coefficients, i.e., hysteresivities, to be 0.7 in the cell body and 0.6 in the lamellipodium, indicating not purely elastic but visco-elastic properties in both regions (Fig. 5) [35]. These coefficients correspond to the power exponents of 0.39 and 0.34 from the structural damping theory. This observation implies that the leading edge behaves partially elastic similar to the cell body but its hysteresivity was not significantly different from the cell body. However, the regional dependence of the storage and loss moduli qualitatively resembles the observation from the optical rheology; the leading edges showed the lower storage and loss moduli than the cell body (Fig. 5), although the quantitative values differ by two orders of magnitude [22]. Similar to the static elastic moduli, this discrepancy may have resulted from the methodological difference.

The regional differences in the static elastic moduli and frequency-dependent viscoelastic moduli with the observations in the structural damping coefficients state that the leading edge of the human neutrophil is softer but still elastic. The lower elastic moduli measured in the leading edge were not dominated by the viscous fluidic behavior but by the elastic behavior. In conclusion, our result is another support that the softer but elastic nature of leading edge of motile neutrophils provides the dynamic fluctuations thereby allowing the active polymerization of the actin filaments to produce the lamellipodial protrusion that leads to the fast locomotion during the immune response [38].

Conclusion

We have investigated the mechanical properties of the human neutrophils using the AFM-based micro-rheology. By adapting the Chen and the Tu models, we were able to determine the viscoelastic behavior of the adherent human neutrophils from the local nano-domains not only in the cell body but also in the thin leading edge. The static nano-indentation measurements conclude that the mechanical behavior of the leading edge and the tail regions were indistinguishable but the cell body displays higher elastic moduli compared the leading edge. The consistent observations were made through the frequency-dependent measurements as well. The elastic and viscous moduli gradually increase from the leading edge to the cell body of the human neutrophils. The structural damping coefficient does not significantly vary locally in a human neutrophil. In conclusion, the leading edge of an adherent neutrophil was observed to be softer but still elastic compared to the cell body. We postulate that this soft, elastic behavior in the leading edge provides the protrusive force for the human neutrophils to carry out the immune responsive reaction.

Acknowledgements

This work was supported in part by the Higher Education Assistance Fund (HEAF) of Texas Tech University.

Conflict of Interests

The authors have declared that no conflict of interest exists.

References

1. Wagner J.G, Roth R.A. Neutrophil migration mechanisms, with an emphasis on the pulmonary vasculature. Pharmacol Rev. 2000;52(3):349-374

2. Muller W.A. Leukocyte-endothelial-cell interactions in leukocyte transmigration and the inflammatory response. Trends Immunol. 2003;24(6):327-334

3. Girard P.P. et al. Cellular chemomechanics at interfaces: sensing, integration and response. Soft Matter. 2007;3(3):307-326

4. Janmey P.A, McCulloch C.A. Cell mechanics: Integrating cell responses to mechanical stimuli. Annu Rev Biomed Eng. 2007;9:1-34

5. Kasza K.E. et al. The cell as a material. Curr Opin Cell Biol. 2007;19(1):101-107

6. Discher D. et al. Biomechanics: Cell Research and Applications for the Next Decade. Ann Biomed Eng. 2009;37(5):847-859

7. Doerschuk C.M. et al. Comparison of Neutrophil and Capillary Diameters and Their Relation to Neutrophil Sequestration in the Lung. J Appl Physiol. 1993;74(6):3040-3045

8. van Eeden S.F. et al. The use of flow cytometry to measure neutrophil function. J Immunol Methods. 1999;232(1-2):23-43

9. Rabodzey A. et al. Mechanical forces induced by the transendothelial migration of human neutrophils. Biophys J. 2008;95(3):1428-1438

10. Perrault C.M. et al. Altered rheology of lymphocytes in the diabetic mouse. Diabetologia. 2004;47(10):1722-1726

11. Lichtman M.A. Rheology of leukocytes, leukocyte suspensions, and blood in leukemia. J Clin Invest. 1973;52:350-358

12. Hochmuth R.M. Micropipette aspiration of living cells. J Biomech. 2000;33(1):15-22

13. Evans E, Yeung A. Apparent Viscosity and Cortical Tension of Blood Granulocytes Determined by Micropipet Aspiration. Biophys J. 1989;56(1):151-160

14. Discher D.E, Mohandas N, Evans E.A. Molecular Maps of Red-Cell Deformation - Hidden Elasticity and in-Situ Connectivity. Science. 1994;266(5187):1032-1035

15. Tsai M.A, Frank R.S, Waugh R.E. Passive Mechanical-Behavior of Human Neutrophils - Effect of Cytochalasin-B. Biophys J. 1994;66(6):2166-2172

16. Henon S. et al. A new determination of the shear modulus of the human erythrocyte membrane using optical tweezers. Biophys J. 1999;76(2):1145-1151

17. Guck J. et al. Optical deformability of soft biological dielectrics. Phys Rev Lett. 2000;84(23):5451-5454

18. Herant M, Heinrich V, Dembo M. Mechanics of neutrophil phagocytosis: experiments and quantitative models. J Cell Sci. 2006;119(9):1903-1913

19. He J.H, Xu W, Zhu L. Analytical model for extracting mechanical properties of a single cell in a tapered micropipette. Appl Phys Lett. 2007;90(2):023901

20. Herant M, Marganski W.A, Dembo M. The mechanics of neutrophils: Synthetic modeling of three experiments. Biophys J. 2003;84(5):3389-3413

21. Heidemann S.R, Wirtz D. Towards a regional approach to cell mechanics. Trends Cell Biol. 2004;14(4):160-166

22. Yanai M. et al. Regional rheological differences in locomoting neutrophils. American Journal of Physiology-Cell Physiology. 2004;287(3):C603-C611

23. Fabry B. et al. Time scale and other invariants of integrative mechanical behavior in living cells. Physical Review E. 2003;68(4):041914

24. Radmacher M. et al. From Molecules to Cells - Imaging Soft Samples with the Atomic Force Microscope. Science. 1992;257(5078):1900-1905

25. Radmacher M, Tilmann R.W, Gaub H.E. Imaging Viscoelasticity by Force Modulation with the Atomic Force Microscope. Biophys J. 1993;64(3):735-742

26. Mahaffy R.E. et al. Scanning probe-based frequency-dependent microrheology of polymer gels and biological cells. Phys Rev Lett. 2000;85(4):880-883

27. Mahaffy R.E. et al. Quantitative analysis of the viscoelastic properties of thin regions of fibroblasts using atomic force microscopy. Biophys J. 2004;86(3):1777-1793

28. Park S. et al. Cell motility and local viscoelasticity of fibroblasts. Biophys J. 2005;89(6):4330-4342

29. Boyum A. Isolation of Mononuclear Cells and Granulocytes from Human Blood - Isolation of Mononuclear Cells by One Centrifugation and of Granulocytes by Combining Centrifugation and Sedimentation at L G. Scandinavian Journal of Clinical & Laboratory Investigation. 1968;21:s77

30. Zeman K, Engelhard H, Sackmann E. Bending Undulations and Elasticity of the Erythrocyte-Membrane - Effects of Cell-Shape and Membrane Organization. Eur Biophys J. 1990;18(4):203-219

31. Guck J. et al. The optical stretcher: A novel laser tool to micromanipulate cells. Biophys J. 2001;81(2):767-784

32. Roca-Cusachs P. et al. Rheology of passive and adhesion-activated neutrophils probed by atomic force microscopy. Biophys J. 2006;91(9):3508-3518

33. Fabry B. et al. Scaling the microrheology of living cells. Phys Rev Lett. 2001;87(14):148102

34. Roca-Cusachs P. et al. Neutrophil microrheology probed by atomic force microscopy. FASEB J. 2006;20(5):A1296-A1296

35. Sollich P. Rheological constitutive equation for a model of soft glassy materials. Physical Review E. 1998;58(1):738-759

36. Ananthakrishnan R. et al. Modelling the structural response of an eukaryotic cell in the optical stretcher. Curr Sci. 2005;88(9):1434-1440

37. Sugitate T. et al. Mechanical role of the nucleus in a cell in terms of elastic modulus. Current Applied Physics. 2009;9(4):E291-E293

38. Mogilner A, Oster G. Force generation by actin polymerization II: The elastic ratchet and tethered filaments. Biophys J. 2003;84(3):1591-1605

39. Goldberg M.B, Theriot J.A. Shigella-Flexneri Surface Protein Icsa Is Sufficient to Direct Actin-Based Motility. Proc Natl Acad Sci U S A. 1995;92(14):6572-6576

40. Drury J.L, Dembo M. Aspiration of human neutrophils: Effects of shear thinning and cortical dissipation. Biophys J. 2001;81(6):3166-3177

Author contact

Corresponding author: phone: 1-806-742-2264, fax: 1-806-742-1182, email: soyeun.parkedu

Received 2010-11-15
Accepted 2011-1-7
Published 2011-1-20

Citation styles

©2024 Ivyspring International Publisher. Terms of use